If you have to find out what the value of x is, then you need to keep the x in order for it to end up equalling to something else
When simplifying the rational expression, explain why it is improper to cancel out the x's. State a general rule for canceling factors in a rational expression and give an example of how this rule would be used?
Get the x alone
Whether it is improper or not depends on the context. If you are simplifying something of the nature of
x(x-3)/(x(x-2))
it might be completely appropriate to cancel the x terms. On the other hand, you should recognize that the function will be undefined at x=0 as well as being undefined at x=2. Even though the function is undefined at x=0, it can still be differentiated and integrated across that point. (The limit exists at that point, so the expression is not indeterminate.) Here is a plot of it. It is identical to the plot of (x-3)/(x-2).
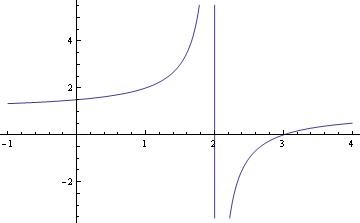
x(x-3)/(x(x-2))
it might be completely appropriate to cancel the x terms. On the other hand, you should recognize that the function will be undefined at x=0 as well as being undefined at x=2. Even though the function is undefined at x=0, it can still be differentiated and integrated across that point. (The limit exists at that point, so the expression is not indeterminate.) Here is a plot of it. It is identical to the plot of (x-3)/(x-2).

