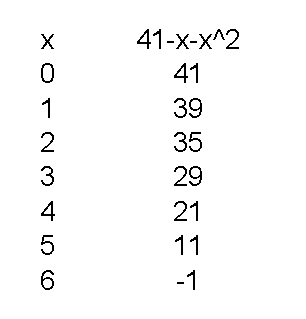Take a look at the picture to see your formula's value for several different x values.
For x=0, N = -(0)2 - (0) + 41 = -0-0+41 = 41.
As it happens, 41 is a prime number. You can check by dividing by odd primes less than 7, that is, 3 or 5.
For x=1, N = -(1)2 - (1) + 41 = -1-1+41 = 39.
39 is a composite number, being the product of 3 and 13.
_____
To check a number for primality, you need only divide by primes up to the square root of the number. 72=49, so all values this formula produces will require testing by only 3 and 5. Any even value other than 2 is not prime. (11, 29, 41 are prime; 21=3*7, 35=5*7, 39=3*13)
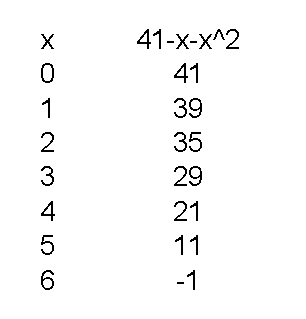
For x=0, N = -(0)2 - (0) + 41 = -0-0+41 = 41.
As it happens, 41 is a prime number. You can check by dividing by odd primes less than 7, that is, 3 or 5.
For x=1, N = -(1)2 - (1) + 41 = -1-1+41 = 39.
39 is a composite number, being the product of 3 and 13.
_____
To check a number for primality, you need only divide by primes up to the square root of the number. 72=49, so all values this formula produces will require testing by only 3 and 5. Any even value other than 2 is not prime. (11, 29, 41 are prime; 21=3*7, 35=5*7, 39=3*13)