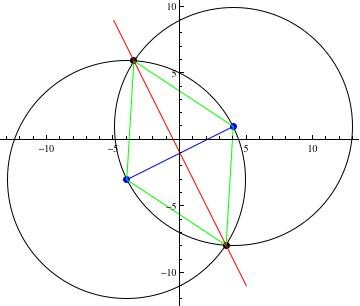
There will be two such vertices. One will be in the second quadrant, and one will be in the fourth quadrant. They will lie on the perpendicular bisector of the line between the given points, and they will be found at the intersection points of circles centered at each point having a radius equal to the distance between the points. (Refer to the figure below.)
The distance between the given points is
r = √((-4-4)^2 + (-3-1)^2) = √(64+16) = √80
The circle centered at (-4, -3) has equation
(x + 4)^2 + (y + 3)^2 = 80
x^2 + 8x + 16 + y^2 + 6y + 9 = 80 (expansion of the equation. Call this [1])
The circle centered at (4, 1) has equation
(x - 4)^2 + (y - 1)^2 = 80
x^2 - 8x + 16 + y^2 - 2y + 1 = 80 (expansion of the equation. Call this [2])
We can set [1] = [2] and solve for y.
x^2 + 8x + 16 + y^2 + 6y + 9 = 80 = x^2 - 8x + 16 + y^2 - 2y + 1
8x + 6y + 9 = -8x - 2y + 1 (subtract x^2+16+y^2 from both sides)
6y = -16x - 2y - 8 (subtract 8x+9 from both sides)
8y = -16x - 8 (add 2y)
y = -2x - 1 (divide by 8. This is the equation of the red line. Call this [3])
We can substitute [3] into [1] and solve for x. In the figure, this gives the x coordinate of the intersection points of the red line and the lower circle.
x^2 + 8x + 16 + (-2x - 1)^2 + 6(-2x - 1) + 9 = 80
x^2 + 8x + 16 + 4x^2 + 4x + 1 - 12x - 6 + 9 = 80 (eliminate parentheses)
5x^2 + 20 = 80 (collect terms)
5x^2 = 60 (subtract 20)
x^2 = 12 (divide by 5)
x = ±2√3 (take the square root)
We can use this result in [3] to find the corresponding values of y. (Positive sign first.)
y = -2(2√3) - 1 = -4√(3) - 1
The quadrant IV vertex is (2√3, -4√(3) - 1).
y = -2(-2√3) - 1 = 4√(3) - 1
The quadrant II vertex is (-2√3, 4√(3) - 1).
The distance between the given points is
r = √((-4-4)^2 + (-3-1)^2) = √(64+16) = √80
The circle centered at (-4, -3) has equation
(x + 4)^2 + (y + 3)^2 = 80
x^2 + 8x + 16 + y^2 + 6y + 9 = 80 (expansion of the equation. Call this [1])
The circle centered at (4, 1) has equation
(x - 4)^2 + (y - 1)^2 = 80
x^2 - 8x + 16 + y^2 - 2y + 1 = 80 (expansion of the equation. Call this [2])
We can set [1] = [2] and solve for y.
x^2 + 8x + 16 + y^2 + 6y + 9 = 80 = x^2 - 8x + 16 + y^2 - 2y + 1
8x + 6y + 9 = -8x - 2y + 1 (subtract x^2+16+y^2 from both sides)
6y = -16x - 2y - 8 (subtract 8x+9 from both sides)
8y = -16x - 8 (add 2y)
y = -2x - 1 (divide by 8. This is the equation of the red line. Call this [3])
We can substitute [3] into [1] and solve for x. In the figure, this gives the x coordinate of the intersection points of the red line and the lower circle.
x^2 + 8x + 16 + (-2x - 1)^2 + 6(-2x - 1) + 9 = 80
x^2 + 8x + 16 + 4x^2 + 4x + 1 - 12x - 6 + 9 = 80 (eliminate parentheses)
5x^2 + 20 = 80 (collect terms)
5x^2 = 60 (subtract 20)
x^2 = 12 (divide by 5)
x = ±2√3 (take the square root)
We can use this result in [3] to find the corresponding values of y. (Positive sign first.)
y = -2(2√3) - 1 = -4√(3) - 1
The quadrant IV vertex is (2√3, -4√(3) - 1).
y = -2(-2√3) - 1 = 4√(3) - 1
The quadrant II vertex is (-2√3, 4√(3) - 1).

