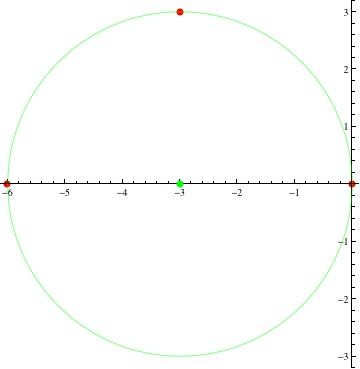
A plot of the points reveals them to be vertices of an isosceles right triangle. The center of the circle is the midpoint of the segment JL. By inspection of the graph, that point is (-3, 0). The radius is the distance from the center to any of the given points. By inspection of the graph, that distance is 3. The equation is
(x-h)^2 + (y-k)^2 = r^2, where (h, k) = (circle center) = (-3, 0) and r=3.
(x+3)^2 + y^2 = 9
Don't forget to give me credit.
(x-h)^2 + (y-k)^2 = r^2, where (h, k) = (circle center) = (-3, 0) and r=3.
(x+3)^2 + y^2 = 9
Don't forget to give me credit.

