How Do You Find The Degree Of A Triangle Using Trigonometry?

HYPOTENUSE : THE SIDE OPPOSITE TO THE RIGHT ANGLE IN A TRIANGLE IS CALLED A

HYPOTENUSE.

I need a formula for calculating the dimensions of 2 sides of a triangle. I know the degrees of all 3 sides of the triangle. 90, 30, 60. The hypotenuse is 36" .
Please help! Anyone?

Similar triangles and proportions

If first angle is x
second angle is 2x
3rd angle is  ???
And all three angles have to add up to the total of all angles of all triangles

Second angle of a triangle is 15 degrees more than the first. The third angle is 23 degrees less than the first. What is the exact measure of all three angles. Help

Well lets say that you have 40 and 50 then you add them up and the answer would be 90 for the last step then you subtract 90 from 180 and 180 because 180 is the triangles length no matter what!

Well you need to know what the angle of a full triangle is.  Then figure out how much of the triangle is missing.  You can subtract those two to find out how much of the angle of the triangle is actually there too using that method.

Each pair of figures is similar. Find the missing side.

180 degrees equals a triangle. If one angle is 30, let's say and another is 70, pretending, then what more is needed to equal 180? In this fake case, it would be 80, which would mean the remaining angle is of 80 degrees.

Use the formula a+b+c=180degrees I had the same question and this is what my teacher told me. It really works I can guarantee that!

You take the highest angle number and subtract the lower number from the higher one therefor your answer is what ever you have subtracted's answer!!! How easy was that?

How do you find the missing angles of a triangle with only one given angle ; the base is 16" and the height is 4"

The measures of the second angle of a triangle is 5 degrees more than 4 times that of the first,and the measure of the third angle is 27 degrees less than three times that of the second angle. Find the measure of each angle.

You can pretty much calculate any angle using trigonometric functions. These functions work with right triangles but will provide answers for any triangle that can be broken into two right triangles.

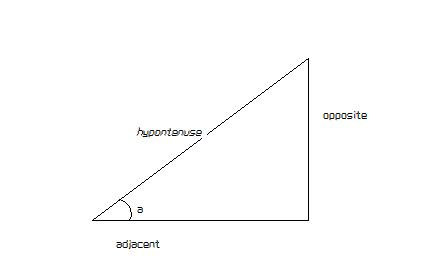
Given the illustration:

The sine of the angle a is a ratio of the length of the opposite side to the length of the hypotenuse.

The cosine of the angle a is a ratio of the length of the adjacent side to the length of the hypotenuse.

The tangent of the angle a is a ratio of the length opposite side to the length of the adjacent side.

Just in case you do not know ratio means divided by.

Knowing the length of any two sides of the triangle we can calculate either the sine, cosine or tangent and from that determine the angle.

Since the sum of the three angles of a triangle must be 180^o and one angle of a right triangle is always 90^o we can calculate the third angle.

As an example suppose the adjacent side is 6 inches and the hypotenuse is 8 inches, we can calculate the cosine of angle a as 6/8 = .750 . If we check the trig tables or a fancy calculator we see that .750 is the cosine of the angle 41.41^o. If you don't have a fancy calculator and you are using the tables you might have to interpolate. (But thats another story).

Given that the unknown angle = 180-(90+41.41) the three angles are 41.41, 90 and 48.59.

There are other trignometric functions, but sine, cosine and tangent will do the bulk of the work.

The three angles are
x
2x
2x + 10
 
They must add up to 180
x + 2x + 2x + 10 = 180
5x + 10 = 180
5x = 170
x = 34 (angle1
 
2x = 68(angle2)
 
y=2x+10
y = 2(34) + 10
y = 78 (angle3)
 
34 + 68 + 78 = 180.
 
 
 

All the angles of a triangle add up to 180 degrees no matter what size the triangle is,  say 1 angle is 50 degrees another is 50 degrees and then whats the last 1? It would have to be 80 degrees --- 50+50+_=180?    50+50+80=180.

Normally in any triangle, the sum of the three angles is 180. you have already know two angles. You have to find the missing angle. Consider triangle  ABC.  In this triangle A, B are the known angles.   We have to find the angle C.  the sum of the three angles is 180. so,          A+B+C =  180,   C = 180 - (A+B)

Your always going to have a length of 180 for your triangle and when you know that everything flows from there , beacuse say your other two angles are 30 and 20 which gives you 50 then you subtract 50 from 180 and get 130 which will be your missing angle ,  I hope this helps in any way :)

There are some formulas which you can use to calculate the degrees of triangle using trigonmetry.

A=angle 1
b = angle 2
c = angle 3
A = side 1
B = side 2
C = side 3

a² = b²+c² - 2bc because A
c² = a² + b² - 2ab because C

Sin A/a =Sin C/c =Sin B/b

Adjacent means next or side by side. Like adjacent angles or adjacent sides. Hypotenuse is the opposite side of 90 degree angle in a right angle triangle. Like , if you draw two lines having right angle, then the third line that connects the two will be hypontenuse.

2592mi=1/2*b*81

You add up the angles given and subtract the sum of those two numbers from 180 degrees because no matter what the triangle will add up to 180... And whatever answer you get is the amount of missing degrees.

And then your done.

I hope this helped.

The 3 angles of a triangle add up to 180 .so if they are x ,y, and z respectively then z=180- x+y